Student seminar: Legendrian contact homology (Fall 2023)
Location: MS 5128
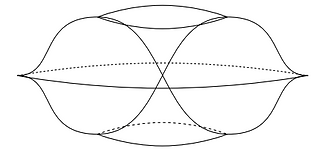
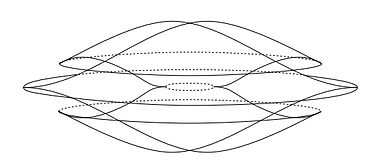
Tentative schedule
Front projections of Legendrian Clifford and Chekanov-Eliashberg tori,
pictures are borrowed from this paper by Georgios Dimitroglou Rizell and Roman Golovko
Abstracts and notes:
Talk 1: Legendrian knots and their invariants.
Abstract: We will give a friendly introduction to Legendrian knots in R^3 and their classical invariants. Noting their limitations, we will also define the more powerful Chekanov-Eliashberg DGA and compute it for a Legendrian trefoil.
Notes for the Talk 1
Talk 2: Chekanov-Eliashberg DGA of Legendrian knots.
Abstract: We finish the definition of the Chekanov-Eliashberg dga, show how to use this to define Legendrian contact homology, and discuss how augmentations give rise to linearized contact homology. Time permitting, we will examine the geometric definition of the Chekanov-Eliashberg dga.
Talk 3: Legendrian contact homology in higher dimensions
Abstract: We will generalize our usual definition of contact structures, Legendrian submanifolds and the related DGA and Legendrian Contact Homology to higher dimensions. We will go through several examples of Legendrian submanifolds in higher dimensions, and the computations of Legendrian Contact Homology.
Talk 4: Infinite families of non-isotopic Legendrian spheres and tori
Abstract: The augmented Chekanov-Eliashberg DGA in higher dimensions is constructed and from this, the linearized contact homology LCH_* is developed. After generalizing some tools from Legendrian knot theory to higher dimensions, it is shown using the LCH that there are Legendrian spheres L_k that lie in pairwise distinct isotopy classes despite having the same classical invariants for n even. Moreover, using a Legendrian suspension, an infinite family of non-isotopic Legendrian tori is presented that share the same classical invariants.
Talk 5: Morse flow trees
Abstract: We will discuss some elementary properties of holomorphic disks and flow trees. Then we will outline a proof on how given certain sequences of J-holomorphic disks into T*M, we can pick a subsequence that converges to a limiting flow tree.
Talk 6: Lagrangian cobordisms between Legendrian knots
Abstract: We give a Morse-theoretic description, via flow trees, of the DGA maps induced by exact Lagrangian cobordisms. We also give combinatorial descriptions of some examples of such maps. We then show an application in finding a lower bound on the number of exact Lagrangian fillings of Legendrian (2,n) torus links.
Notes for the Talk 6
Talk 7: Microsupport and constructible sheaves
Abstract: We will briefly discuss some basics of sheaf theory and six-functor formalism concentrating mostly on relevant examples. We will then introduce the singular support (microsupport) of a sheaf on manifold and compute microsupports in several toy examples. Time permitting, I will speculate about the relationship between the category of sheaves with singular support in a given Legendrian knot and the augmentations of the associated Chekanov-Eliashberg dga.
Notes for the Talk 7
Talk 8: Microlocal sheaves on Legendrians and Lagrangian cobordisms
Abstract: Sheaves with microsupports on Legendrians (with fixed microlocal rank) can be viewed as representations of the Legendrian contact dg algebra with coefficients enriched over the fundamental group. We will explain the microlocalization or microlocal monodromy functor which produces a local system (a microsheaf) on the Legendrian from the sheaf on the manifold, analogous to the fundamental group coefficient in the Legendrian contact dg algebra. Then we will explain functors between sheaves and (representations of) Legendrian contact dg algebra induced by Lagrangian cobordisms, whose full faithfulness induces long exact sequences.
Notes for the Talk 8
Talk 9: Surgery formulas for linearized contact homology and symplectic homology
Abstract: In this talk we will explore surgery formulae for Chekanov-Eliashberg algebras of Legendrian submanifolds in a fillable contact manifold. Applications to this Legendrian surgery formula include computations of Chekanov-Eliashberg algebras of some Legendrian knots, contact homology algebras of certain contact manifolds, and symplectic invariants of the corresponding symplectic filling.
Notes for the Talk 9
Talk 10: Contact homology and 1-parameter families of Legendrian knots
Abstract: We will discuss 1-parameter families of Legendrian knots in the standard contact R3. Using the monodromy invariant in Legendrian contact homology, we will show that there is an ‘exotic’ S1-family of Legendrian right-handed trefoils, which is not contractible through S1-families of Legendrian but contractible through S1-families of smooth knots.
Talk 11: Towards understanding of the relationship between Lagrangian Floer theory and Chekanov-Elishberg dga
Abstract: Given a Lagrangian submanifold L in a Weinstein domain X with Legendrian boundary \Lambda in the contact boundary of X, it is natural to ask whether invariants of L, such as Lagrangian Floer homology, have an explicit interpretation in terms of invariants of \Lambda. In this talk, I will show that for a subcritical Weinstein domain X, Chekanov-Eliashberg dga of \Lambda is isomorphic to the cobar construction associated with A_\infty-algebra CF^*(L). One of the key steps is an isomorphism between the two setups of Chekanov-Eliashberg dga: one via loop space coefficients and the other in terms of parallel copies. Time permitting I will speculate about the extension of the above result to the general case in the context of surgery formulas.
Notes for the Talk 11

Adrien-Marie Legendre
References:
[1] Asplund, Johan, and Tobias Ekholm. ”Chekanov-Eliashberg dg-algebras for singular Legendrians.” arXiv preprint arXiv:2102.04858 (2021).
[2] Bourgeois, Fr ́ed ́eric, Tobias Ekholm, and Yakov Eliashberg. ”Effect of Legendrian surgery.” Geometry & Topology 16.1 (2012): 301-389.
[3] Georgios Dimitroglou Rizell. Lifting pseudo-holomorphic polygons to the symplectisation of P × R and applications. Quantum Topol., 7(1):29–105, 2016.
[4] Ekholm, Tobias, John Etnyre, and Michael Sullivan. ”The contact homology of Legendrian submanifolds in R2n+1.” Journal of Differential Geometry 71.2 (2005): 177-305.
[5] Ekholm, Tobias, John Etnyre, and Michael Sullivan. ”Non-isotopic Legendrian submanifolds in R2n+1.” Journal of Differential Geometry 71.1 (2005): 85-128.
[6] Ekholm, Tobias, John Etnyre, and Michael Sullivan. ”Orientations in Legendrian contact homology and exact Lagrangian immersions.” International Journal of Mathematics 16.05
(2005): 453-532.
[7] Ekholm, Tobias. ”Morse flow trees and Legendrian contact homology in 1–jet spaces.” Geometry & Topology 11.2 (2007): 1083-1224.
[8] Ekholm, Tobias. ”Rational SFT, linearized Legendrian contact homology, and Lagrangian Floer cohomology.” Perspectives in Analysis, Geometry, and Topology: On the Occasion of the 60th Birthday of Oleg Viro. Boston, MA: Birkh ̈auser Boston, 2011. 109-145.
[9] Ekholm, Tobias, Ko Honda, and Tam ́as K ́alm ́an. ”Legendrian knots and exact Lagrangian cobordisms.” Journal of the European Mathematical Society (EMS Publishing) 18.11 (2016).
[10] Ekholm, Tobias, and Yanki Lekili. ”Duality between Lagrangian and Legendrian invariants.” arXiv preprint arXiv:1701.01284 (2017).
[11] Etnyre, John B., and Lenhard L. Ng. ”Legendrian contact homology in R3.” arXiv preprint arXiv:1811.10966 (2018).
[12] Etnyre, John B., Lenhard L. Ng, and Joshua M. Sabloff. "Invariants of Legendrian knots and coherent orientations." (2002): 321-367.
[13] Ghiggini, Paolo. ”Introduction to Legendrian knots and Legendrian contact homology.”
(2012).
[14] Honda, Ko. ''3-dimensional contact geometry course notes".
[15] Kashiwara, M., and Schapira, P. (2013). Sheaves on Manifolds: With a Short History. Les d ́ebuts de la th ́eorie des faisceaux. By Christian Houzel (Vol. 292). Springer Science Business Media.
[16] Ng, L., Rutherford, D., Shende, V., Sivek, S., and Zaslow, E. (2020). Augmentations are sheaves. Geometry & Topology, 24(5), 2149-2286.
[17] Shende, Vivek, David Treumann, and Eric Zaslow. ”Legendrian knots and constructible sheaves.” Inventiones mathematicae 207 (2017): 1031–1133.
[18] Pan, Yu. "Exact Lagrangian fillings of Legendrian (2, n) torus links." Pacific Journal of Mathematics 289.2 (2017): 417-441.
[19] Chantraine, Baptiste, et al. "Floer theory for Lagrangian cobordisms." Journal of differential geometry 114.3 (2020): 393-465.
[20] Li, Wenyuan. "Lagrangian cobordism functor in microlocal sheaf theory I." Journal of Topology 16.3 (2023): 1113-1166.
[21] Kálmán, Tamás. "Contact homology and one parameter families of Legendrian knots." Geometry & Topology 9.4 (2005): 2013-2078.